Qualifiers to the World Cup Russia 2018: An introduction to the theory of probability

November 15^th, 2017

Argentina, Colombia, and Peru qualified to the World Cup Russia 2018. The latter qualified beating New Zealanad 2-0 in the interconfederation play-off.

October 7^th, 2017

In the first part of this article, published on September 11^th, we elaborated a mathematical analysis to predict the Conmebol teams that will qualify to the World Cup Russia 2018. The analysis was done before Matchday 17 and Matchday 18, we assumed that Colombia was not going to lose against Paraguay, but for ironies of soccer Paraguay defeated Colombia, scoring 2 goals in the last 5 minutes. This outcome radically changed the results discussed in the analysis. We considered a positive result of Colombia because, by doing so, the possible combinations were reduced from 2187 to 243 (refer to the first part of the article), the latter being an easy-to-handle number. In this second part, we are going to develop a similar analysis considering the results of Matchday 17. The standings are as follow:

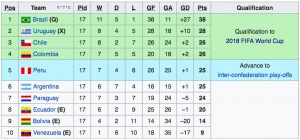
To begin with, we are going to consider that in Matchday 18, Uruguay vs Bolivia is an irrelevant game; therefore, we have 4 games left, giving us:

• Possible combinations in Matchday 18: 3 x 3 x 3 x 3 = 81.

This means that there are 81 ways in which the standings can be left (click here to see the 81 possible ways). Of all of them only one will represent the end of the Qualifiers. In this analysis we have two qualified teams: Brazil and Uruguay. The candidates for the other three places are Chile, Argentina, Peru, Colombia, and Paraguay (in this article, a “qualified team” includes the team that advances to the inter-confederation play-offs). Their games are as follow:

———– vs Argentina
Peru vs Colombia 
———– vs Chile
Paraguay vs ———–

As it can be seen, in Matchday 18 it does not matter against which teams Argentina, Chile, and Paraguay play because the only ones who can alter the standings are them and not their rivals. Now we will use the theory of probability…

Based in mathematics we can answer the following questions:

1.How many points is likely to have the last qualified team?
Considering the tree diagram (explained in the first part of this article) we obtain:

P(28) = 6/81
P(27) = 27/81
P(26) = 42/81
P(25) = 6/81

P(X) indicates the probability of obtaining X points in the last qualifying spot. Therefore, it is likely that the 5^th place in the Conmebol Qualifiers will have 26 points, P(26) = 42/81 = 51.9%. Additionally, we see that the highest score possible is 28 points. Uruguay to date has 28 points with a goal difference of +10; consequently, to be eliminated her rivals must win 9-0 at least. In these circumstances, it is feasible to assume that Uruguay is already qualified.

2. In how many cases the last qualified team and the best eliminated team are tied in points?

Using the results given in the supplementary information we have:

TOTAL PROBABILITY: 27/81 = 33.3%

PROBABILITY (If Brazil beats Chile) = 15/27 = 55.5%
PROBABILITY (If Chile ties Brazil) = 6/27 = 22.2%
PROBABILITY (Si Chile beats Brasil) = 6/27 = 22.2%

This means that in 27 cases out of 81, the 5^th and 6^th place in the standings are settled by goal difference. For the particular case in which Chile loses against Brazil, this situation happens in 15 out of 27 cases.

3. Which teams will qualify?

In this analysis, Brazil and Uruguay are already qualified. There are 3 spots left to reach the World Cup. Based on the results shown in the supplementary information we have for the teams that are still competing:

The interesting thing is that although Peru and Argentina have the same amount of points before playing their matches, once they finish them, the qualifying probability of Argentina is higher. The reason is because Peru plays against a direct rival that is Colombia. For the analysis of Paraguay we omitted the case with best goal difference because for this to happen Paraguay must thrash Venezuela 7-0. If these results would be relevant, the 3 qualified teams would be Chile, Colombia, and Argentina.
However, it is important to note that although Paraguay has the lowest probability, she has the most accessible game, facing Venezuela in Asuncion. In contrast, although Chile has the best qualifying probability, she faces Brazil in Sao Paulo. Therefore, we can perform a similar analysis taking these data into account. That is to say, if we assume that Paraguay beats Venezuela, and Chile as much ties Brazil, the qualifying probability becomes:

From the Table above we can conclude the following:

• Paraguay, beating Venezuela 1-0 reaches a qualifying probability of 83.3%.
• Chile is the second team most likely to qualify; nevertheless, her qualifying probability strongly depends in goal difference (goes from 55.6% to 83.3%). The advantage that Chile has with respect to the other teams is that even if losing against Brazil her qualifying probability remains latent. A curious fact that can be seen by paying attention to the supplementary information is that for Chile, a draw or a win against Brazil gives the same outcome, that is, it does not modify her qualifying probability nor it harms the other teams. With this, Chile could calmly play defense against Brazil.
• Colombia is the third team with more chances to qualify, her qualifying probability remains similar with best or worst goal difference. Colombia has the advantage that if she ties Peru, her chances to qualify remain latent. That is, Colombia could calmly play defense against Peru.
• Argentina and Peru have the lowest qualifying probabilities, they must win their matches so as to not depend on many variables. Therefore, they must play offense in Matchday 18.

Finally, it is worth mentioning that the unexpected triumph of Paraguay greatly benefited Chile and seriously damaged Peru, as evidenced by comparing these calculations with those made in the first part of the article… Who will take their songs to the World Cup? Will Argentina and Peru give the surprise? Will Paraguay lose her great option? Will Chile, being favorite, be eliminated? Or Colombia, being third, will remember her match against Paraguay and realize that they lost their chances to qualify in the 5 last minutes? On October 10^th, everything will be revealed…

Christian Ortiz, PhD.